Advanced Math Solutions – Integral Calculator, advanced trigonometric functions, Part II

In the previous post we covered integrals involving powers of sine and cosine, we now continue with integrals involving powers of secants and tangent of the form \tan^n(x)\sec^m(x).

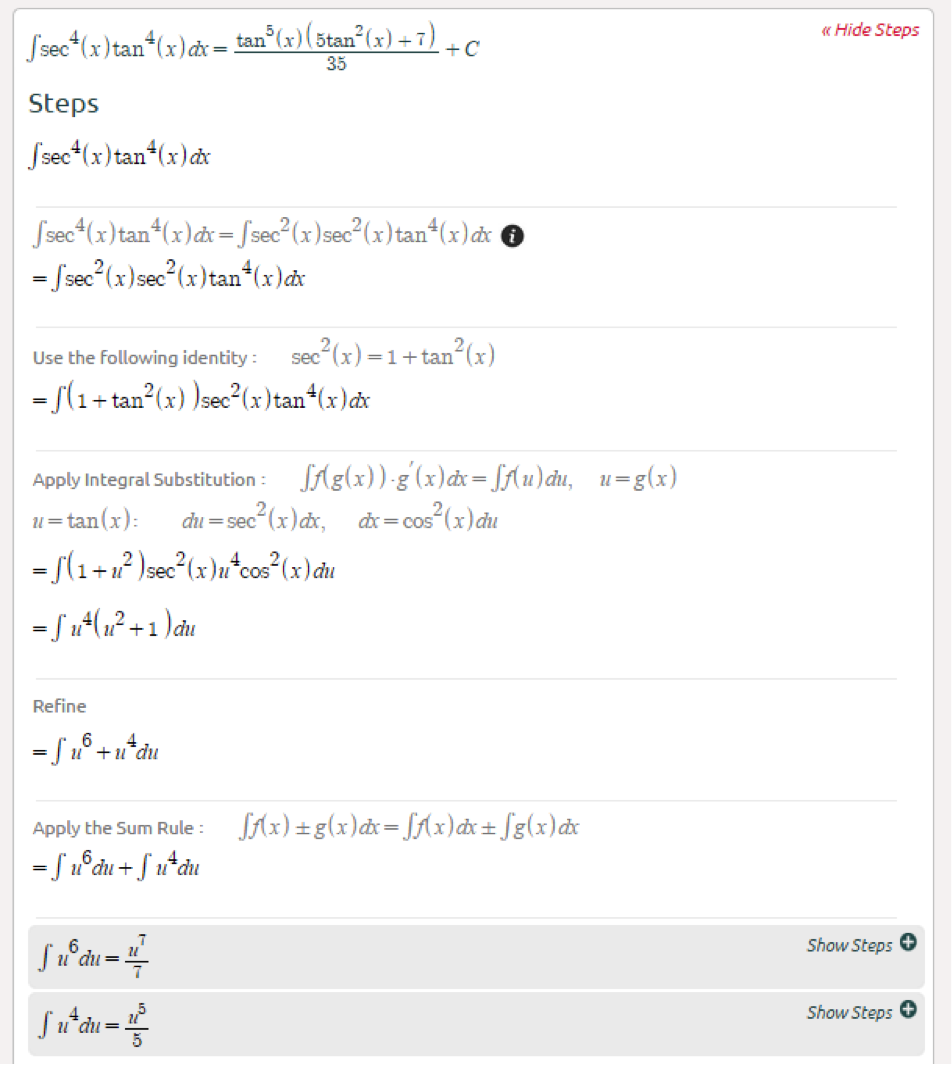
The general rule to evaluate \int \tan^n\left(x\right)\sec^m\left(x\right)dx is as follows:

• If m is even and positive, take \sec^2(x) out, use the identity \sec^2(x)=1+\tan^2(x) to convert remaining sec to tan, and substitute u=\tan(x)
• If n is odd and positive, take \tan(x)\sec(x) out, use the identity \tan^2(x)=\sec^2(x)-1 to convert remaining tan to sec, and substitute u=\sec(x)
• If n is odd or even but there is no \sec(x) factor, use the identity \tan^2(x)=\sec^2(x)-1 and repeat the steps above
• If none of the cases apply, try converting to sine and cosine

Let’s see how it works starting with an example where m is even (click here):

Simply combine the solutions and substitute back u=\tan(x).

Here’s another example where n is odd (click here):

One more example where n is odd with no factor of sec(x) (click here):

In the next post we’ll cover trigonometric substitution, stay tuned.


Cheers,
Michal