High School Math Solutions – Trigonometry Calculator, Trig Simplification

Trig simplification can be a little tricky. You are given a statement and must simplify it to its simplest form. The problem is that sometimes you don’t even know where to begin. Your eyes are glued to the problem, your head begins to hurt, and you keep writing the same equation over and over. I’ve been in that position before and hope to help make sure that never happens again. In this blog post, we will go over very helpful trig identities and some examples of trig simplification.

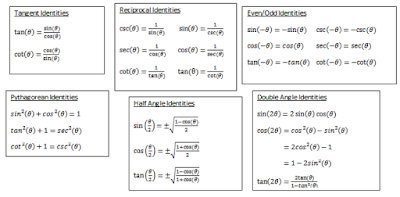
Here are just a couple trig identities to memorize:

Although I haven’t listed them, memorizing algebraic properties, like how to expand or factor polynomials, will be helpful too.

There is no exact formula you can follow to simplify these functions. That is why I won’t be going over a problem step by step. However, I will give you some tips on how to approach these problems.

Tips & Tricks:

1. Look for obvious simplifications when you begin
2. See if you can factor anything
3. Get rid of fractions
4. If trig functions are multiplied together, see if you can rewrite one or both
5. See what trig functions you can rewrite
6. Memorize the identities
7. Practice makes perfect
8. Keep your work nice and neat

Now that you have some of my tips and tricks in mind, let’s see some examples.

First example (click here):

I can’t see any immediate simplifications when I begin, but I know that I can rewrite \cos^2(\theta). By doing that, it opens the function up to be simplified.

Next example (click here):

In this problem, there are two fractions. I want to get rid of them, so I combine them into one fraction. By doing that, I immediately see I can rewrite the numerator. There are now two different trig functions multiplied together in the denominator. I want to rewrite one or both of the functions, but I remember an identity and rewrite both.

Sometimes your first try of simplifying might go awry. Don’t give up! Try again. It may be frustrating, but the more practice and experience you get with these problems, the better you will become at trig simplification. Remember my tips and tricks and it should make your life a little easier.

Until next time,
Leah

Advanced Math Solutions – Limits Calculator, Advanced Limits

In our previous posts we have gone over multiple ways of solving limits. In this post we will talk about advanced limits that not only require certain methods we have talked about like, L’Hopital, squeeze theorem, etc., but also require application of properties and the algebraic limit theorem.
Here are some properties you will find very helpful when finding limits:

We won’t go each problem step by step because each problem is different from the other, but we will have two examples to show different types of advanced limits that use multiple ways to solve them.


Here’s the first example (click here):

In this example we use the algebraic property and the algebraic limit theorem to get the function in a form, where we can apply L’Hopital’s rule and find the limit.



Here’s the last example (click here):

In this example, we use the algebraic limit theorem to get the function in a form where we can apply the squeeze theorem and find the limit.

In order to solve these advanced limits it is important that the different methods and properties are fresh on your mind. Since there is no exact recipe on how to solve each advanced limit problem because each problem is different, I will tell you what I found easiest for me.


Leah’s Advice:

1. Memorize all the different ways to solve a function, such as substitution, factoring, L’Hopital, the chain rule, etc. 
• I recommend  making flash cards
• It will be a pain to keep flipping through your notes to remember what each method is
2. Practice the methods
• Practice problems for each method until you are a pro at solving them
• This goes along with memorization, you want to memorize how use each method 
• Practice makes perfect!

Until next time,

Leah