High School Math Solutions – Absolute Value Equation Calculator

Solving absolute value equations is somewhat tricky; it requires understanding of the absolute value property.

In short, to solve simple absolute value equations first isolate the absolute value on one side of the equation, numbers on the other side.  Write two simple equations without absolute values like this:  |f(x)|=a  =>  f(x)=a  or  f(x)=-a

Finally, solve the two simple equations.  (click here for a quick review of simple equations)

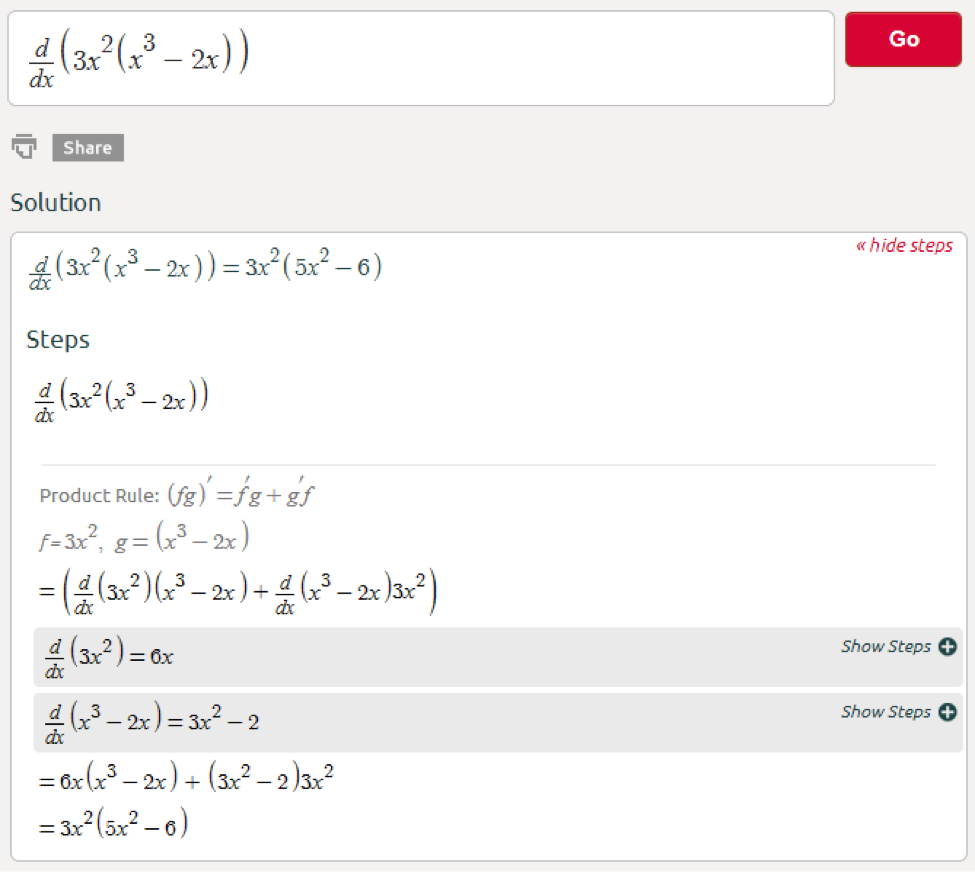
Here's how it works (click here):

Solving equations involving multiple absolute values or variables requires some more attention.

Here you should first find the positive and negative ranges of the absolute values.  Write the simple equations for the different ranges, solve and validate the solutions are within the range.

Here's how it works (click here):

Until next time,
Michal

High School Math Solutions – Derivative Calculator, the Chain Rule

In the previous posts we covered the basic derivative rules, trigonometric functions, logarithms and exponents (click here).  But we are still missing the most important rule dealing with compound functions, the chain rule.

Why is it so important?  Because most of the functions you will have to derive, and later integrate, are most likely compound.  For example sin(2x) is the composition of f(x)=sin(x) and g(x)=2x or √(x²-3x) is the composition of f(x)=√x and g(x)= x²-3x

The chain rule formula is as follows:  (f(g(x)))’=f’(g(x)) *g’(x)
That is, the derivative of the composition of two functions equals the derivative of the outer function times the derivative of the inner function


Let’s start with an example to see how it works (click here):

Here’s a more complex example involving multiple applications of the chain rule (click here):

With the chain rule we put it all together; you should be able to derive almost any function.  There are some advanced topics to cover including inverse trig functions, implicit differentiation, higher order derivatives, and partial derivatives, but that’s for later.

Until next time,
Michal

High School Math Solutions – Derivative Calculator, Logarithms & Exponents

In the previous post we covered trigonometric functions derivatives (click here). We can continue to logarithms and exponents.  The derivatives of the natural logarithm and natural exponential function are quite simple. The derivative of ln(x) is 1/x, and the derivative of e^x is, e^x.

From these, we can use the logarithms and exponents rules to differentiate the generalized form of logarithmic or exponential functions, or just get familiar with these four derivatives:

(ln(x))' = 1/x
(e^x)' = e^x
(a^x)' = a^x ln(a)
(log_a(x))’ = 1/x ln(a)

Here’s how it works, (click here):

Here’s another example (click here):

Until next time,
Michal

High School Math Solutions – Derivative Calculator, Trigonometric Functions

In the previous posts we covered the basic algebraic derivative rules (click here to see previous post). But how can we derive trigonometric functions?  Simply by memorizing some common trig derivatives:

(sin(x))’ = cos(x)

(cos(x))’=-sin(x)

(tan(x))’=sec²(x)


Let’s start with an example, (click here):

Here’s another example (click here):

Until next time,
Michal

High School Math Solutions – Derivative Calculator, Products & Quotients

In the previous post we covered the basic derivative rules (click here to see previous post).  We are now going to step up a bit to differentiate products & quotients.  Functions involving products and quotients seem more complex, but once you follow the derivative rules it’s straightforward. Start by identifying the different components (i.e. multipliers and divisors), derive each component separately, carefully set the rule formula, and simplify.

Product rule:   (fg)’ = f’g + g’f

Quotient rule:  (f/g)'=(f'g+g'f)/g^2


Let’s start with an example using the product rule, (click here):

Here’s an example using the quotient rule (click here):

Until next time,
Michal

High School Math Solutions – Derivative Calculator, the Basics

Differentiation is a method to calculate the rate of change (or the slope at a point on the graph); we will not differentiate using the definition which requires some tricky work with limits; but instead we will use derivative rules that are fairly easy to memorize. We’ll start with the basics:

Constant:  (c)’=0
Power rule:  (xⁿ)’=nx^(n-1)
Multiplication by constant:  (cf(x))’=c(f(x))’
Sum/difference rule:  (f±g)’=f’± g’

We are good to go.

Let’s start with an example, click here to see how it works

Here’s another example (click here):

Until next time,
Michal